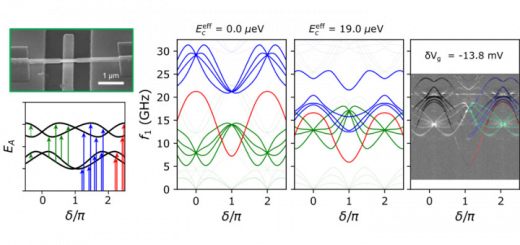Charge and spin transfer statistics of quantum impurity models

Tuesday, 15 July 2009, 12:00-13.00

Dr. Andreas Komnik

Institut für Theoretische Physik, Universität Heidelberg

ABSTRACT:

We analyze the full counting statistics (FCS) of the charge and spin transport through the Anderson impurity model (AIM) and similar systems with a single conducting channel. The object of principal interest is the generating function for the cumulants of charge current distribution. We derive an exact analytic formula relating the FCS generating function to the self energy of the system in the presence of the measuring field. We first check that our approach reproduces correctly known results in simple limits, such as the FCS of the resonant level system (AIM without Coulomb
interaction) then proceed to study the FCS for the AIM both perturbatively in the Coulomb interaction and in the Kondo regime at the Toulouse point as well as around the its fixed point. At zero temperature the FCS turns out to be binomial for small voltages. For the generic case of arbitrary energy scales the FCS is shown to be captured very well by generalizations of the Levitov-Lesovik type formula.
Surprisingly, the FCS for the AIM indicates a presence of coherent electron pair tunnelling in addition to conventional single-particle processes. By means of perturbative expansions around the Toulouse point we succeeded in showing the universality of the binomial FCS at zero temperature in linear response. Based on our general formula for the FCS we then argue for a more general binomial theorem stating that the linear response zero-temperature FCS for any interacting single-channel setup is always binomial.