Periodic Energy Transport and Entropy in Quantum Electronics
Title: Periodic Energy Transport and Entropy in Quantum Electronics.
When: Monday, July 2, (2018), 12:00.
Place: Department of Theoretical Condensed Matter Physics, Faculty of Sciences, Module 5, Seminar Room (5th Floor).
Speaker: David Sánchez, Institute for Cross-Disciplinary Physics and Complex Systems IFISC (UIB-CSIC), Palma de Mallorca, Spain.
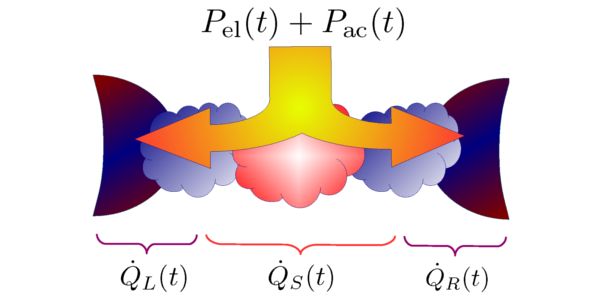
Most of the recent literature on quantum thermodynamics focuses on static fields and the resulting stationary transport effects. However, there is a growing interest in analyzing thermodynamic properties of quantum conductors in the presence of time-dependent potentials. In this case, dynamics is the main objective of the theory as fluxes and responses depend explicitly on time. It is also of paramount importance for potential applications to discriminate which portion of the energy invested to operate quantum devices is amenable to be used and which one is wasted by dissipation. This distinction is at the heart of thermodynamics and is conventionally addressed in quasistatic processes where the system under study is very weakly coupled to the reservoirs. In quantum electronics, nevertheless, the generic situation is to have the driven structure strongly coupled to the rest of the circuit, which plays the role of a reservoir.
Here, I will discuss in detail the energy transfer through a mesoscopic conductor attached to fermionic reservoirs. The energies of the sample evolve with time due to the coupling with nearby ac gate terminals. Deep inside the reservoirs, electrons relax their excess energy and the baths can thus be considered in local thermal equilibrium. We will also consider the entropy production in the whole system and will identify the different terms arising in the redistributed energy and heat. Importantly, when the energies shift slowly with time the response is adiabatic and an exact Joule law can be demonstrated for the time domain. Our analysis is completely general and does not rely on the particular approach followed to evaluate the relevant dynamical quantities.